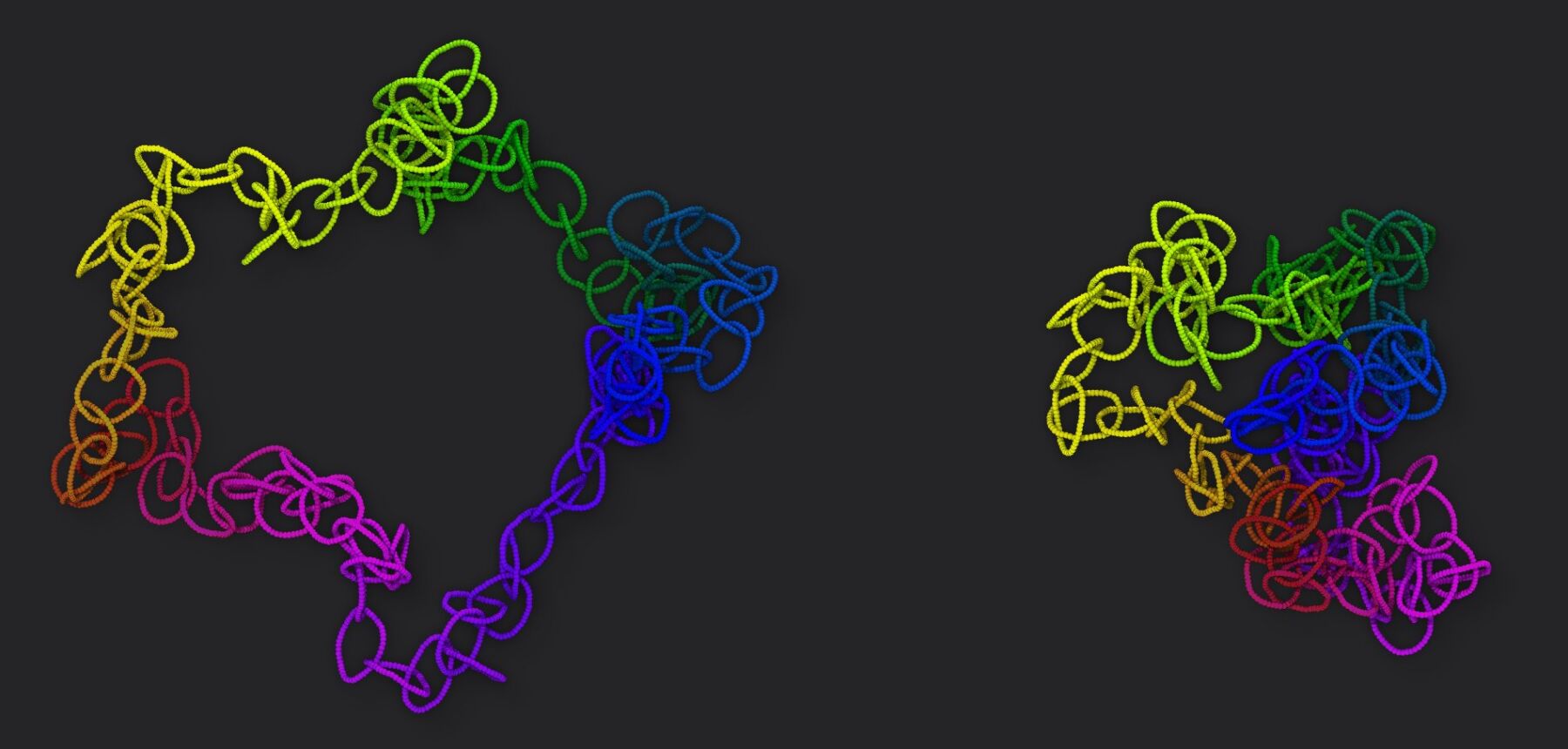
Macrochains of topologically interlocked rings with unique physical properties have recently gained considerable interest in supramolecular chemistry, biology, and soft matter. Most of the work has been, so far, focused on linear chains and on their variety of conformational properties compared to standard polymers. Here we go beyond the linear case and show that, by circularizing such macrochains, one can exploit the topology of the local interlockings to store torsional stress in the system, altering significantly its metric and local properties. Moreover, by properly defining the twist (Tw) and writhe (Wr) of these macrorings we show the validity of a relation equivalent to the Cǎlugǎreanu-White-Fuller theorem Tw+Wr=const, originally proved for ribbon like structures such as ds-DNA. Our results suggest that circular structures of topologically linked rings with storable and tunable torsion can form a new category of highly designable multiscale structures with potential applications in supramolecular chemistry and material science

Download “Article preprint” Ring_o_rings.pdf – Downloaded 116 times – 11 MB

Download a copy of the manuscript (preprint version)