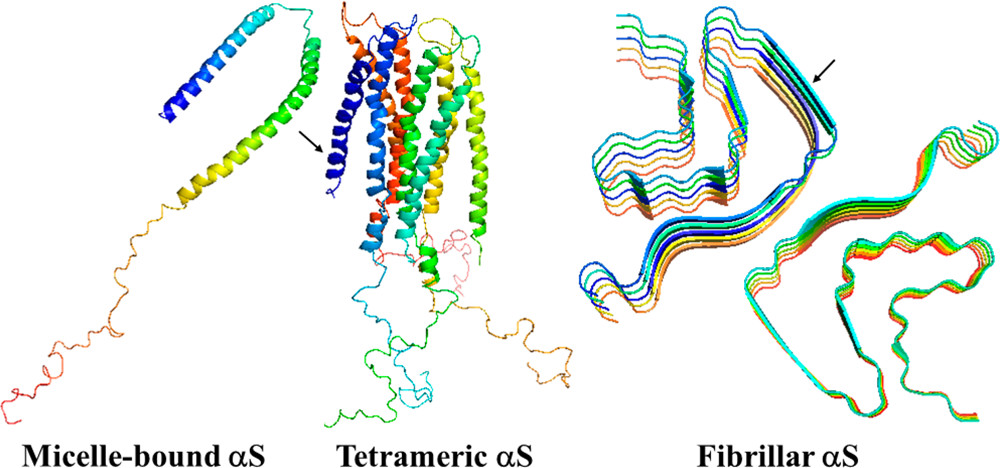
α-Synuclein (αS) is the principal protein component of the Lewy body and Lewy neurite deposits that are found in the brains of the victims of one of the most prevalent neurodegenerative disorders, Parkinson’s disease. αS can be qualified as a chameleon protein because of the large number of different conformations that it is able to adopt: it is disordered under physiological conditions in solution, in equilibrium with a minor α-helical tetrameric form in the cytoplasm, and is α-helical when bound to a cell membrane. Also, in vitro, αS forms polymorphic amyloid fibrils with unique arrangements of cross-β-sheet motifs. Therefore, it is of interest to elucidate the origins of the structural flexibility of αS and what makes αS stable in different conformations. We address these questions here by analyzing the experimental structures of the micelle-bound, tetrameric, and fibrillar αS in terms of a kink (heteroclinic standing wave solution) of a generalized discrete nonlinear Schrödinger equation. It is illustrated that without molecular dynamics simulations the kinks are capable of identifying the key residues causing structural flexibility of αS. Also, the stability of the experimental structures of αS is investigated by simulating heating/cooling trajectories using the Glauber algorithm. The findings are consistent with experiments.
Download a copy of the manuscript