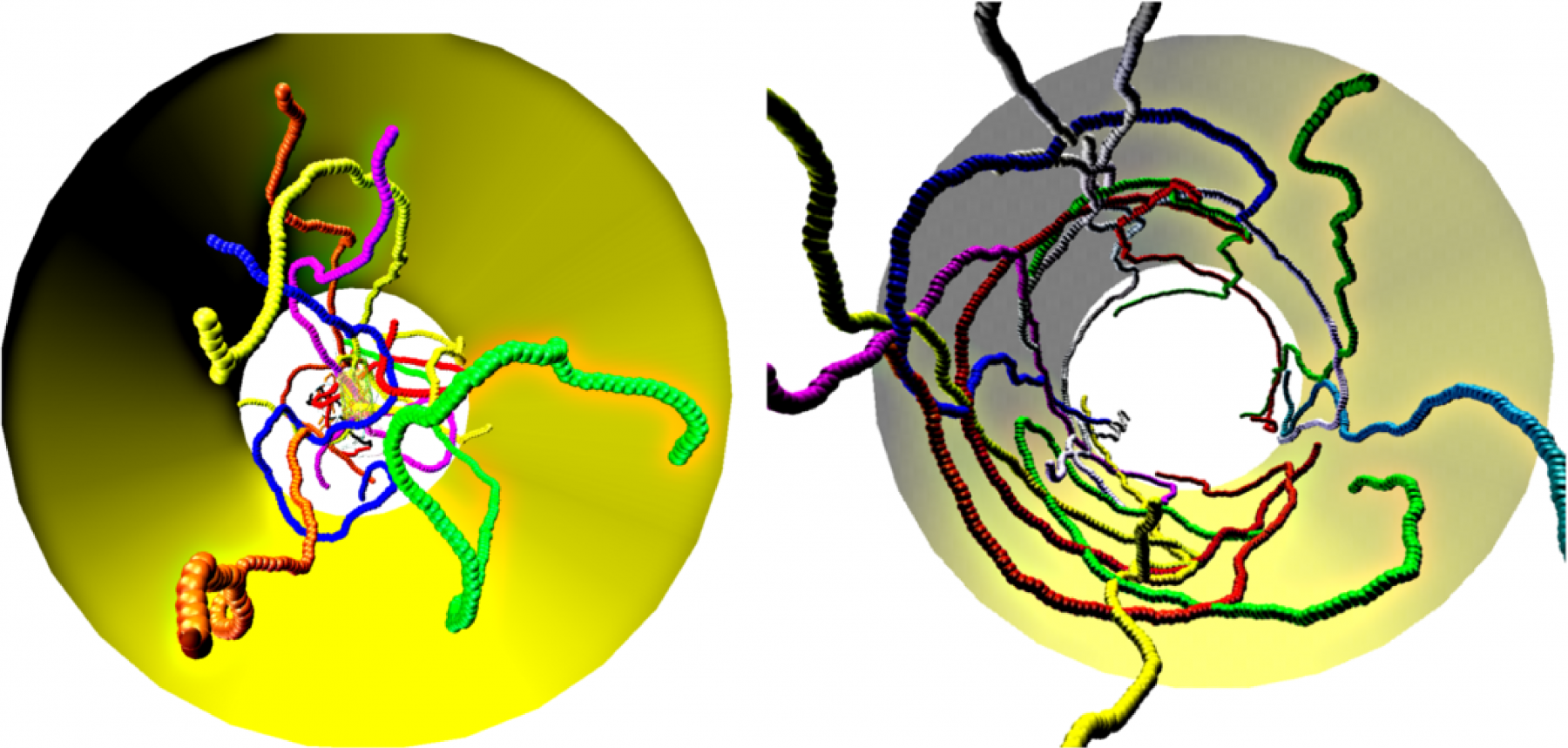
Conformations of wormlike chains in cylindrical pores with attractive walls are explored for varying pore radius and strength of the attractive wall potential by molecular dynamics simulations of a coarse-grained model. Local quantities such as the fraction of monomeric units bound to the surface and the bond-orientational order parameter as well as the radial density distribution are studied, as well as the global chain extensions parallel to the cylinder axis and perpendicular to the cylinder surface. A nonmonotonic convergence of these properties to their counterparts for adsorption on a planar substrate is observed due to the conflict between pore surface curvature and chain stiffness. Also the interpretation of partially adsorbed chains in terms of trains, loops, and tails is discussed.
Download a copy of the manuscript (preprint version)